the NCERT solutions for class 5 maths chapter 7 are a great way to improve your mathematical skills.With tips and tricks provided in these solutions, you can excel in mathematics effortlessly.So don’t hesitate to use them as they will help you achieve academic excellence!
Students, let’s delve into the step-by-step solutions for Class Five Maths Chapter Seven.Firstly, we must acknowledge that these solutions are comprehensive and cater to all levels of understanding.Each solution is succinctly explained and easy to follow, with helpful diagrams and examples provided throughout.The solutions cover a wide range of topics such as numbers in words, addition, subtraction, multiplication and division – where every problem has been approached systematically to ensure a clear understanding
NCERT Solutions for Class 5 Maths Chapter 7 Can You See the Pattern? has been published by exammodalpaper. You can now download the Class 5 Maths Ch 7 Questions and Answers PDF here. This NCERT Solutions for Class 5 Maths contains answers of all questions asked in Chapter 7 in textbook. Therefore you can prerefer it to solve Be My Multiple, I’ll be Your Factor exercise questions and learn more about the topic.In lesson 7 of Class 5 Maths, students learn .
These materials are prepared as per the CBSE and ncert syllabus (2023-2024) formulated for Class 5. Maths is an important subject, and it is always crucial to have a thorough understanding of the basics. These elementary concepts form the base for higher education and also shape future opportunities. The solved questions provided here follow the Class 5 NCERT Textbook curriculum.
NCERT Solutions for class 5 Maths chapter 7 Can You See the Pattern?
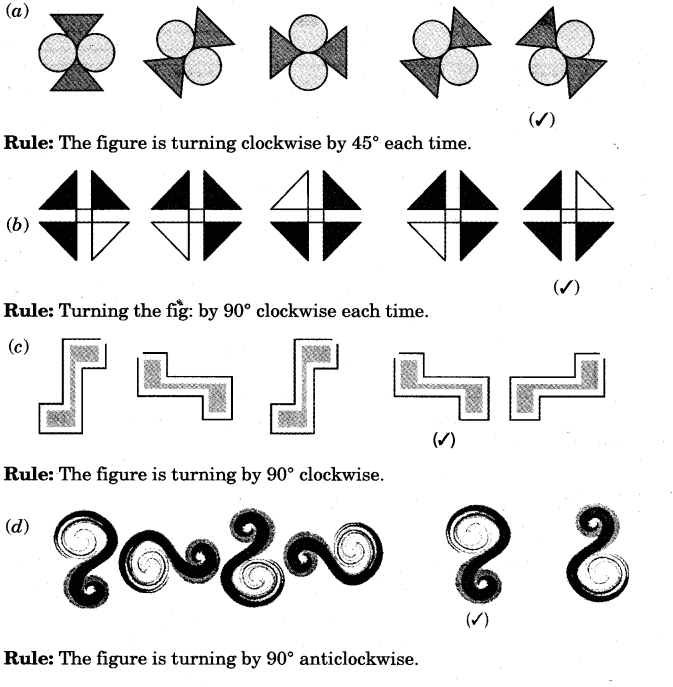
Pattern 1

1.

Ans.In pattern 1, we can see clearly that the rule is: one up, one down. Then this is repeated.
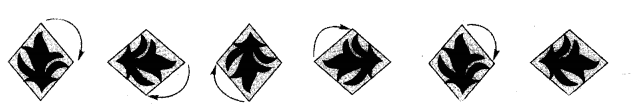
In pattern II, the rule is: it is once up, then takes a 1/4 turn every time. The rule is to repeat it with 1/4 clockwise turn.

In pattern III, the rule is : 1/4 turn anti-clockwise.

Practice Time
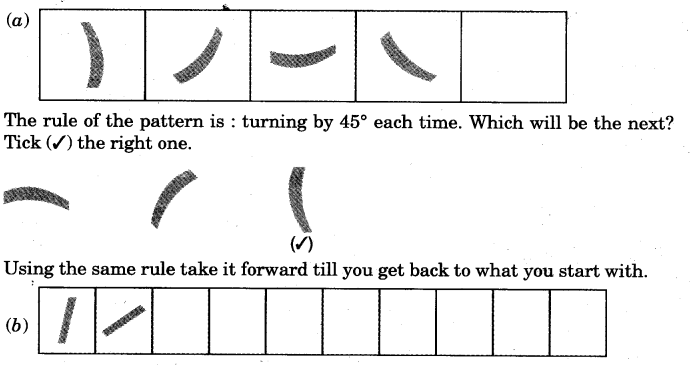
1.What should come next?
Ans. Answer figures are given in last two boxes.
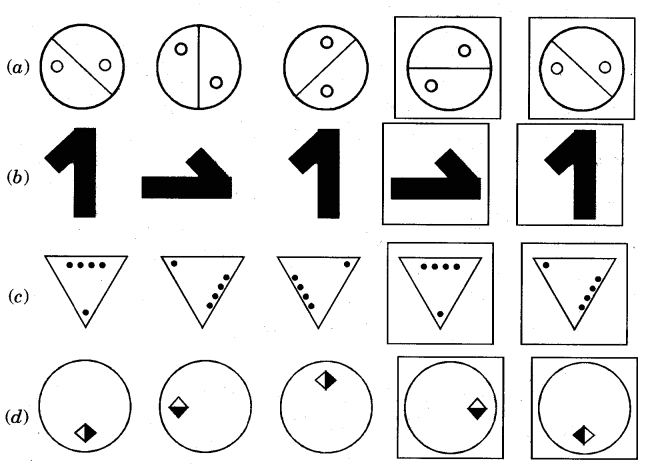
2.See this Pattern
Ans.
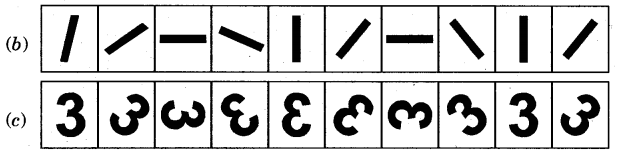
3.Some patterns are given below on the left side of the red line. For each pattern, write the rule. Then choose what comes next from the right side of the line and tick (right) it.
Ans.
Look for a Pattern
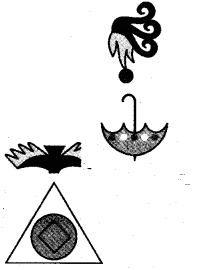
1.Mark that picture which is breaking the rule. Also correct it.
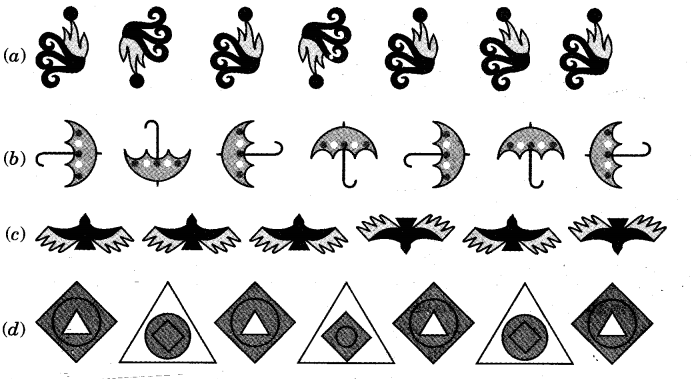
Ans. (a) In this pattern the sixth figure from the left is breaking the rule.
Correct figure is as follows:
(b)The sixth figure from left is breaking the rule.
(c)The second figure from left is breaking the rule. Correct figure is as follows:
(d)The fourth figure from left is breaking the rule. Correct figure is as follows:
Magic Squares
Do you remember magic triangles? Come now, let’s make some magic squares.
1.Fill this square using all the numbers from 46 to 54.
Rule: The total of each line is 150
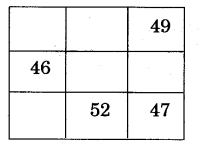
Ans. In the third row, we have
52 + 47 =99
And 150-99 =51
So, we can fill 51 in the first box of the third row.
Now, in the first column, we have 46 + 51 =97
And 150-97 =53
So, we can fill 53 in the first box of the first column.
In the first row, we have
53 + 49 = 102
And 150 – 102 = 48
So, we can fill 48 in the second box of the first row.
In the second column, We have
48 + 52 =100
And 150-100 =50
So, we can fill 50 in the second box of the second column. In the third row, we have
49 + 47 =96
And 150 – 96 = 54
So, we can fill 54 in the second box of the third column.
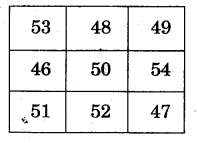
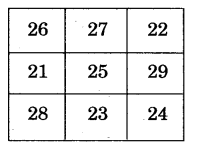
2.Fill this square using all the numbers from 21 to 29. Rule: The total of each side is 75.
Ans. total of each line is equal to 75.
Hence, the complete magic square is as follows:
Magic Hexagons
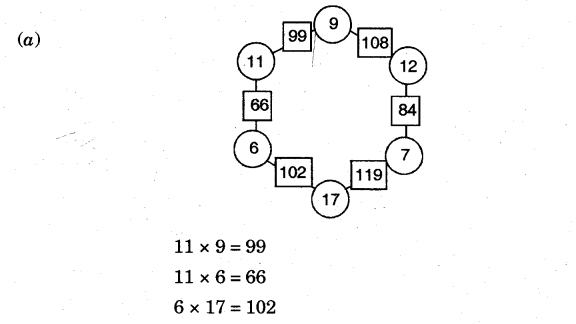
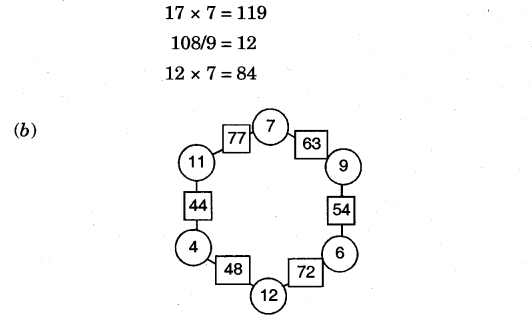
1.Leek at the patterns of numbers in hexagons. Each side has 2 circles and 1 box. You get the number in each box by multiplying the numbers in the circles next to it.
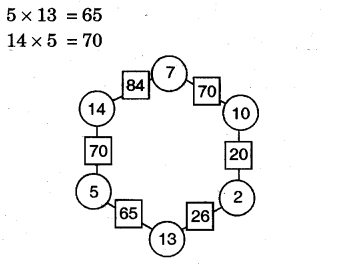
Look at the number 65 in the box.
Which are the circles next to it?
Can you see how tite rule works?
Use the same rule to fill the hexagons below:
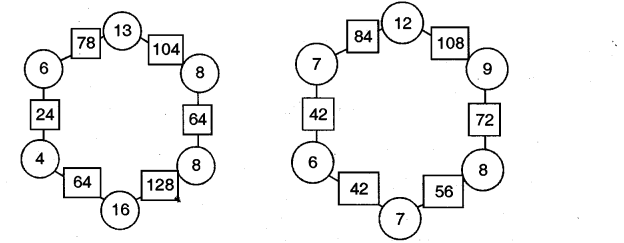
2.Now you also make your own magic hexagons.
Ans. Some of the magic hexagons are given below:
Numbers and Numbers
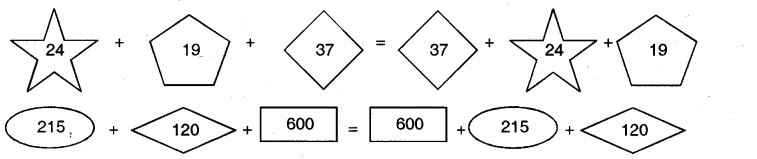
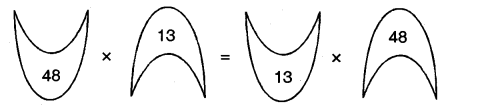
1.Are they equal?
Ans.Yes they are equal.
2.Fill in the blank spaces in the same way.
Ans.
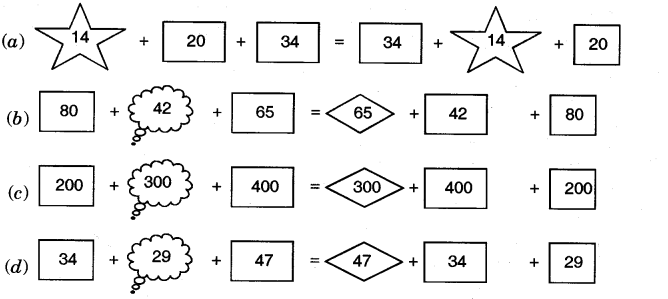
3.Now look this
Ans.
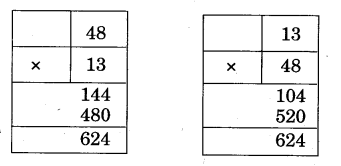
Left Right—Same to Same
1.Can you see something special about 121?
Ans. The number reads the same in backward and forward.
2.What, it’s just a number!
Ans. Oh, yes! It is 1, 2, 1 from right to left also.
Take a number, say : 43
Now turn it back to front : 34
Then add them together : 77
77 is one such special number. There are many such numbers. Take another number : 48
Now turn it back to front : 84
Then add them together : 132
3.Is this a special number? No! Why not?
Ans. Because it is not the same after reversing it.
OK, carry on with the number : 132
Again turn it back.to front : 231
Then add the two together : 363
Ah 1 363 is a special number.
Ans. Yes, it is a special number.
So we see that to get special numbers we sometime need more steps. Now you try and change these numbers into special numbers:
(а)28
Ans. 82
110 This is not a special number; so let us do more steps Oil
121 This is a special number
(b)132
Ans. 231
363 This is a special number
(c) 273
Ans. 372
645 This is not a special number; so let us do more steps 546
1191 This is not a special number; so let us do more steps 1911
3102 This is not a special number; so let us do more steps 2013
5115 This is a special number
4.Now let’s use words in special way.
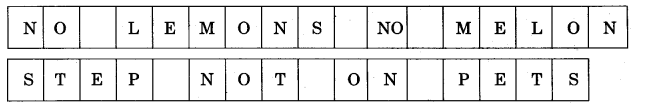
Did you notice that it reads the same from both sides; right to left and left to right?
Now try and use words in a special way.
Ans. Some such examples are given below:
BACON NO CAB
BAR CANOE ON A CRAB
DENNIS SINNED
EVIL OLIVE
Look at the calendar below:
Let us mark a 3 x 3 box (9 dates) on the calendar and see some magic
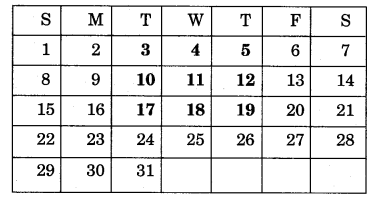
Take the smallest number:3
Add 8 to it: +8
= 11
Multiply by 9:x 9
Total: 99
99 is equal to the sum of all these numbers.
Now you choose any 3×3 box from a calendar and find the total in the same way. Play this game with your family.
Let us take 4
Add 8 to it +8
= 12
Multiply it by 9
= 9 x 12 = 108
This is equal to the sum of these 9 numbers.
To get the answer even faster multiply the middle number by 9, i.e. 12 x 9 = 108.
12 x 2 + 3 = 27
12 x 3 + 3 = 39
12 x 4 + 3 = 51
12 x 5 + 3 =63
12 x + 3 =—————-
———–x 7 + 3 =————
Ans.12 x 6 + 3 = 75
12 x 7 + 3 = 87
12 x 8 + 3 =99
12 x 9 + 3 =111 .
In every case the difference between consecutive numbers is 12
2.Now try doing it with some other number and also take a different number to add at each step.
Ans. Let us take another number to see the pattern;
15 x 2 + 5 =35
15 x 3 + 5 = 50
15 x 4 + 5 =65
15 x 5 + 5 =80
15 x 6 + 5 = 95
15 x 7 + 5 = 110
15 x 8 + 5 = 125
We can see the difference between two subsequent numbers is 15
3.Look at the numbers below. Look for the pattern. Can you take it forward?
(9-1) ÷8 =1
(98 – 2) ÷8 = 12
(987 – 3) ÷8 = 123
(9876 – 4) ÷8 = ………….
(98765-4) ÷8 = …………
(…………. -………….. ) ÷8 =………….
(…………….. -…………)÷8 = ……………
Ans.The above pattern is not correct. The correct pattern is as follows:
(9876 – 4) ÷8 = 1234
(98765-5)÷8 =12345
(987654 – 6) ÷8 =123456
(9876543 – 7) ÷8 =1234567
Smart Adding
1.What if someone gives you to add ten numbers together?
Ans. Multiply 10/2 by (10 + 1) = 5 x 11 = 55
1 + 2 + 3 + 4 + 5 + 6 + 7 + 8 + 9 + 10 =55
11 + 12 + .. .. + .. .. + .. .. + .. .. + .. .. + .. .. + .. .. + 20 =155
21 + .. .. + .. .. + .. .. + .. .. + .. .. + .. ,. + .. .. + .. .. + 30 = ….
31 + .. .. + ., .. + .. .. + .. .. + .. .. + .. .. + .. .. + ., .. + 40 =….
41 + .. .. + ., .. + .. .. + .. .. + .. .. + .. .. + .. .. + .. .. + 50 = ….
51 + .. .. + ., .. + .. .. + .. .. + .. , . + . , .. + .. .. + .. .. + 60 =555
61 + .. .. + .. .. + .. .. + .. .. + .. .. + .. .. + .. .. + .. .. + 70 = ….
2.Did you notice some pattern in the answers?
Ans. Yes, I have noticed some pattern; sum of 1 to 10 is 55, sum of 11 to 20 is 155, sum of 21 to 30 is 255 and so on.
1 + 2 + 3 + 4 + 5 + 6 + 7 + 8 + 9 + 10 =55 11 + 12 + 13 + 14 + 15 + 16 + 17 + 18 + 19 + 20 = 155
21 + 22 + 23 + 24 + 25 + 26 + 27 + 28 + 29 + 30 = 255
31 + 32 + 33 + 34 + 35 + 36 + 37 + 38 + 39 + 40 = 355
41 + 42 + 43 + 44 + 45 + 46 + 47 + 48 + 49 + 50 = 455
51+ 52 + 53 + 54 + 55 + 56 + 57 + 58 + 59 + 60 = 555
61 + 62 + 63 + 64 + 65 + 66 + 67 + 68 + 69 + 70 = 655
Fun with Odd Numbers
Take the first two odd numbers. Now add them, see what you get.
Now, at every step, add the next odd number.
1 + 3 = 4 =2 x 2
1+3+5=9=3 x 3
1 + 3 + 5 + 7 = 16 =4 x 4
l+3 + 5 + 7 + 9 = … =… x …
1 + 3 + 5 + 7 + 9 + 11 = …=… x ….
1 + 3 + 5 + 7 + 9 + 11 + 13 = … = … x …
1.How far can you go on?
Ans. Complete patterns are as follows:
1+3 + 5 + 7 + 9 = 25=5 x 5
1 + 3 + 5 + 7 + 9 + 11 = 36 = 6 x 6
1 + 3 + 5 + 7 + 9 + 11 + 13 = 49 =7 x 7
This process can go on and on.
The sum of first ‘n’ odd numbers can be given by ‘n x n’
Secret Numbers
Banno and Binod were playing a guessing game by writing clues about a secret number. Each tried to guess the other’s secret number from the clues.
1. Can you guess their secret numbers?
Ans.
- It is larger than half of 100.
- It is more than 6 tens and less than 7 tens.
- The tens digit is one more than the ones digit.
- Together the digits have a sum of 11.
2.What is my secret number?
Ans. Let us find this number.
- It is larger than half of 100 means > 50.
- It is more than 6 tens and less than 7 tens means the number is between 60 and 70.
- The tens digit is one more than the ones digit means ones digit is 6 – 1 = 5.
- Hence the number is 65.
- It also means that sum of the digits is 11.
3.It is smaller than half of 100.
It is more than 4 tens and less than 5 tens.
The tens digit is two more than the ones digit.
Together the digits have a sum of 6.
What is my secret number?
Ans. The number is less than 50 and more than 40. Hence the tens digit is 4 and ones digit is 6 – 4 = 2. The secret number is 42.
4.Write a set of clues for a secret number of your own. Then give it to a friend to guess your secret number.
Ans. A set of clues to find the secret number are as follows:
- It is larger than half of 100.
- It is more than 6 tens and less than 7 tens.
- The tens digit is two less than the ones digit.
- The sum of the digits is 14.
- Find the secret number.
- It is smaller than half of 100.
- It is more than 3 tens and less than 4 tens.
- The digit sum is 10.
- Find the secret number.
Number Surprises
(a) Ask your friend—Write down your age. Add 5 to it. Multiply the sum by 2.
Subtract 10 from it. Next divide it by 2. What do you get?
Is your friend surprised?
Ans. Age = 9
Add 5 to it: 9 + 5 = 14
Multiply the sum by 2: 2 x 14 = 28
Subtract 10 from it: 28 – 10 = 18
Divide it by 2: 18÷2 = 9
The answer is same as my friend’s age. So, he must be surprised.
(b) Take a number = ….
Double it: …. x 2 = ….
Multiply by 5: …. x 5 = ….
Divide your answer by 10: …. ÷10 = …
Ans. Let us take the number 9
9 x 2 = 18
18 x 5 = 90
90 ÷10 = 9
Which is equal to the number taken.
(c)Take a number =…
Double it: … x 2 = …
Again double it: …. x 2 = ….
Add the number you took first to the answer Now again double it: …. x 2 = …
Divide by 10: …. ÷10 = …
Ans. Let us take the number 8
8 x 2 = 16
16 x 2 = 32
32 + 8 = 40
40 x 2 = 80
80÷10 = 8
Which is same as the number taken.
(d)Look at this pattern of numbers and take it forward
1 = 1 x 1
121= 11 x 11
12321 =111x 111
1234321 = ?
Ans. The pattern of numbers hints at following answer: 1234321=1111x 1111 Similarly, 123454321 = 11111 x 11111
2.Now make your own number surprises.
Ans. Some examples are as follows:
Take a number = …
Multiply by 4 : … x 4 = …
Add the number taken first: + … = ….
Divide by 5: ….÷5 = …
Let us take 11
11 x 4=44
44 + 11=55
55 ÷5 = 11
Related Post :-
Chapter 4. Parts and Wholes
Chapter 5. Does it Look the Same?
Chapter 6. Be My Multiple, I’ll be Your Factor
Chapter 7. Can You See the Pattern?
Chapter 8. Mapping Your Way
Chapter 9. Boxes and Sketches
Chapter 10. Tenths and Hundredths
Chapter 11. Area and its Boundary
Chapter 12. Smart Charts
Chapter 13. Ways to Multiply and Divide
Chapter 14. How Big? How Heavy?
NCERT Solutions Class 5 to 10
- NCERT Solutions for class 5 Maths
- NCERT Solutions for class 5 EVS
- NCERT Solutions for class 5 Paryayana Adyayan
- NCERT Solutions for class 5 English
- NCERT Solutions for class 5 Hindi
Frequently Asked Questions For NCERT Solutions for class 5 Maths chapter 7 Can You See the Pattern?
Q1: What is the title of Class 5 Maths Chapter 7?
A: The title of Chapter 7 is “Can You See the Pattern?”
Q2: What does this chapter cover?
A: This chapter covers the concept of patterns in mathematics.
Q3: What are NCERT solutions?
A: NCERT solutions are the answers and explanations provided for the questions and exercises given in the NCERT textbooks. They are designed to help students understand and solve problems effectively.
Q4: Where can I find NCERT solutions for Class 5 Maths Chapter 7?
A: You can find NCERT solutions for Class 5 Maths Chapter 7, “Can You See the Pattern?”, in the official NCERT textbooks or on various educational websites and online platforms that provide study materials for students.
Q5: Why are NCERT solutions important?
A: NCERT solutions serve as a useful resource for students as they provide step-by-step explanations and solutions to the exercises.
Q6: How can NCERT solutions for Class 5 Maths Chapter 7 benefit me?
A: NCERT solutions for Class 5 Maths Chapter 7 can benefit you by providing a clear understanding of patterns in mathematics. They help you recognize and analyze patterns effectively and solve problems related to patterns.
Q7: Are NCERT solutions for Class 5 Maths Chapter 7 available online?
A: Yes, NCERT solutions for Class 5 Maths Chapter 7 are available online on various educational websites, educational apps, and online platforms that offer study materials for students.
Q8: Can I rely solely on NCERT solutions for Class 5 Maths Chapter 7 for exam preparation?
A: While NCERT solutions are a valuable resource, it is recommended to refer to the NCERT textbook and additional reference materials to have a comprehensive understanding of the subject. Practice solving a variety of problems to strengthen your knowledge and prepare well for exams.
Q9: Are NCERT solutions for Class 5 Maths Chapter 7 free to access?
A: Yes, many websites and platforms offer free access to NCERT solutions for Class 5 Maths Chapter 7. However, some platforms may require a subscription or payment for accessing certain features or advanced materials.
Q10: Are NCERT solutions for Class 5 Maths Chapter 7 available in languages other than English?
A: Yes, NCERT solutions for Class 5 Maths Chapter 7 may be available in languages other than English, depending on the educational platform or website you are using. Some platforms offer solutions in regional languages for better understanding by students.
